Once in a while, I get interested in a math problem and spend a lot of time working on it. Lately, some such problems have come to my attention via Twitter. But I also enjoy being a participant in a workshop, e.g. at a conference, or in a Math Teachers Circle, as that makes it possible to work with other math teachers on the problem.
I've also been a presenter at Math Teacher Circles in the Bay Area. In fact, this Saturday, March 4, I'll lead a workshop in San Francisco, on geoboard problems for teachers, titled "Connect the Dots". Read about it here, and join us if you can!
(You might also consider signing up for one or both of my summer workshops. See below!)
Meanwhile, here is my newsletter.

Blog Posts
Here are links to posts on my Math Education Blog that you might find interesting.
If you are so moved, you may comment on the posts, and/or subscribe to the blog.
Comparing Two Approaches
In this post, I compare two hypothetical approaches to teaching about the area of a trapezoid. In the traditional approach, students are given a formula, and asked to apply it many times. In the approach I favor, students are guided towards a discovery of the formula. Part of my goal with the post was to make clear that the latter approach does not rule out a teacher explanation. Quite the opposite: it makes it possible for the students to understand a teacher explanation. The post generated interesting comments. Find it here.
Algebra Manipulatives
The Lab Gear is the algebra manipulative I designed in the 1990's. It was based in part on studying the strengths and weaknesses of existing designs, including the well-known and inexpensive Algebra Tiles. But I also much expanded the applications and user-friendliness of algebra manipulatives. Several teachers who have used both tiles and Lab Gear have assured me that the Lab Gear is much more popular among students. Just as importantly, it is supported by much better curricular materials. (Sorry about the self-promotion, but it's true! For a technical comparison of the various algebra manipulative models, click here.)
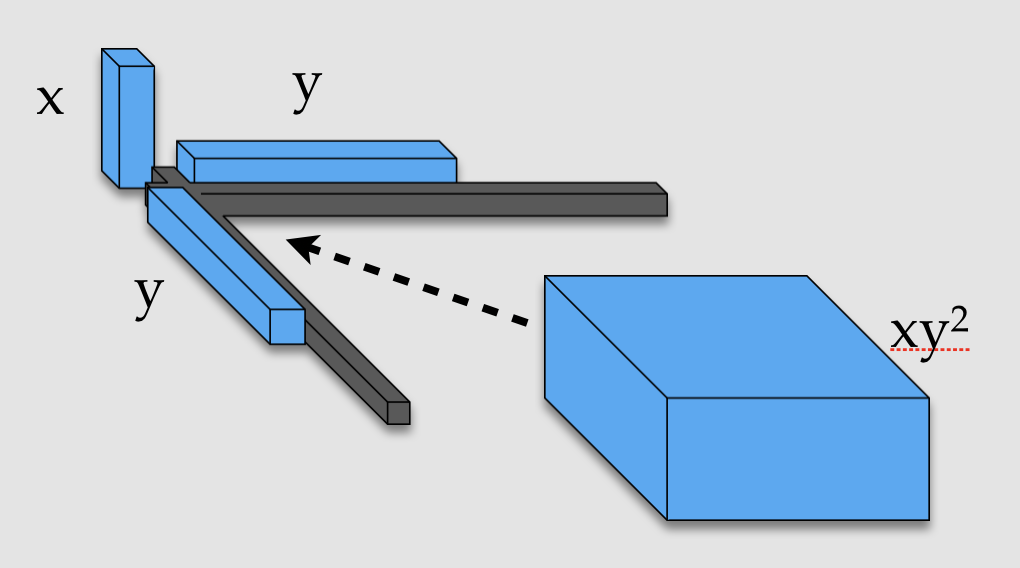
A correspondent asks about the thinking behind the sequencing of the concepts in the Lab Gear books. It's a good question, especially since the sequencing is different in the "basic algebra" vs. the "Algebra 1" version of the materials. I try to answer this question here.
Why Homework
Periodically, I come across the suggestion that homework should be abolished. The argument usually comes from thoughtful educators who realize that in fact, most learning happens at school. I don't disagree with that premise, but in many if not most classes, there is a role for homework. In my classes, collaboration is the default, and help is just about always available from classmates or the teacher. Homework is a chance for students to see what they can do on their own. And in fact, many, many students over the years have told me that going over homework with their neighbors was key to their learning. But what about students whose home situation makes it difficult or impossible to do homework? I address that question here.
MathEducation.page
Vist my Web site!
π Day Is Coming Up!
Here are some possibly useful links for π Day.
- If we accept that the circumference of a circle is 2πr, we can deduce the area formula with the help of this applet.
- Is there a "π" for regular polygons? Use these slides for a discussion in cases that do not require trigonometry. Follow up by using basic trig in the last activity of Geometry Labs (Lab 11.8).
- Learn about taxicab geometry in Geometry Labs 9.1 and 9.6. The latter includes a discussion of "taxi-π". (Note: this is more than one period's worth of work! But it is motivating and interesting.)
Geometric Puzzles in the Classroom
Geometric puzzles change the dynamic in the classroom. As students work on them, they are focused, patient, persistent — many attitudes that are essential to doing math. Puzzles also allow different students to shine. One drawback is that they tend to be solitary endeavors, and usually don't lend themselves to collaboration. Still they offer many opportunities to learn math, and have been a part of my teaching repertoire for over 40 years. Read more about this, find links, and download PDFs here.
Navigating the Site
For a one-person Web site, my MathEducation.page is huge: more than 300 pages, and almost 600 PDFs. It's probably not that easy to find what you're looking for. I put what might be the most useful links right on the front page. In addition, I created three site maps:
- An incomplete, annotated map organized by topics
- An incomplete map organized by grade level and courses
- A nearly-complete compact, comprehensive, and redundant map, organized alphabetically
I link to all three maps in a navigation box at the top and bottom of almost every page on the site. The navigation box also includes direct links to various important places on the site: just hover over the tiny symbols on the left and right sides.
If all else fails, or before using any of these approaches, you can find a Search link in the navigation box. I used to pay Google a yearly fee to run the search, but they just notified me that starting soon, the search box will be accompanied by advertising. I am looking for an alternative. If you know of a good way to offer ad-free search on my site, let me know!