Math Education Newsletter

Here are links to posts on my Math Education Blog, and to items on my Math Education Page.

Transformational Proof

I have taught transformational geometry for decades in my Space course (a post-Algebra 2 elective.) More recently, to address the Common Core State Standards for Math suggestion that geometric transformations be introduced in 8th grade, I developed some materials for that, which you can find on either my Middle School page, or my Transformational Geometry page.

What was missing was a transformational approach to the high school geometry course. The few attempts I have seen were not impressive, and may well be the reason that many geometry teachers think that a transformational approach is "not rigorous". Meanwhile, some of the professor-written materials on this did not turn out to be all that helpful. So, with my colleague Lew Douglas, I embarked on a project to fill that hole in the curriculum.

After a few years of working on this off and on, we have finally completed Phase One of this project: we have worked out the underlying mathematical and logical framework for a transformational approach to many key topics in the traditional geometry course, starting with a small list of axioms, and going on to transformational proofs of theorems about congruence, special triangles and quadrilaterals, similarity, and more. We hope our 45-page booklet on this will be useful to teachers and curriculum developers.

Read my recent blog post about this, and check out plenty of related material on my Web site.

Geometric Construction

This is a topic I have blogged about many times.

In a recent installment, I try to get to the bottom of this mathematically, and I share the strategy that has worked well for me in the classroom. The key is to avoid the "recipe" approach ("This is how you construct a perpendicular bisector. Now practice.") Instead, present construction challenges as puzzles ("Given this line segment, find points that are equidistant from its endpoints. Now construct its perpendicular bisector.") Electronic tools, such as GeoGebra, help a lot.

I have also enjoyed Euclidea, a geometric construction Web site and app. I wrote about it here.

Geometric Puzzles

Many students at all grade levels enjoy geometric puzzles. I have built a fair amount of curriculum (grades 5-10) around tangrams, pentominoes, and supertangrams. Last spring, I received an e-mail from a teacher who had asked her students to do some creative work using geometric puzzles. One student found the artistic potential of the polyarcs, which are my own invention, and are also discussed on my Web site. I share the student's colorful images, and some thoughts about geometric puzzles, in this blog post.

Taxicab Geometry

In taxicab geometry, distance is measured not as the "shortest path", but by moving only parallel to the axes. So for example the taxicab distance from (0,0) to (3,4) is 7. I have for many years included a short unit on this topic in my geometry class, prior to work involving the usual (Euclidean) distance. One discussion-generating fun fact is that a "taxi-circle" is actually a Euclidean square. (A taxi-circle is the set of points equidistant from a center point, using taxicab distance.) I shared these lessons in Geometry Labs 9.1 and 9.6.

In a recent Math Teachers' Circle workshop, I extended this to an exploration of points equidistant from two points, as well as taxi-ellipses, taxi-hyperbolas, and taxi-parabolas. Read about it in this blog post. If you want to explore taxicab geometry on your own, you can download the worksheet. While it is intended for teachers, it may well provide an excellent companion to a precalculus unit on conics.

New and not-so-new on my Web site.

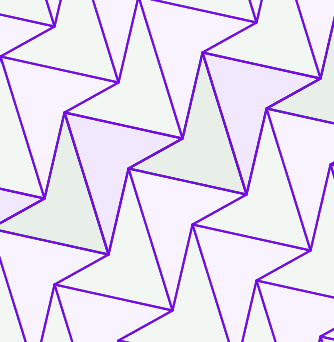
Tiling

One thing that occurred to me this summer was that over the years, I had developed quite a few activities about tiling (aka tessellation) for grades 5-12. I decided to make them all accessible from a Tiling home page. Go there if you are looking for engaging middle school or high school activities involving angle basics, geometric transformations and symmetry, or Archimedean tilings. (The latter offer a good way to preview Archimedean solids.)

Errata

Many students fear math because they feel it's a subject where the teacher is constantly looking for your mistakes. Certainly, we should be looking for ways to build from student strengths, but it is important to make clear to them that mistakes are an inevitable part of learning, and in fact that math teachers and curriculum developers make plenty of mistakes.

I am no exception! If you combine my mistakes with the ones made by my publishers, there is no shortage of needed corrections. I have put some of those on my Web site, and will put more on as I find out about them. Here are links to the corrections:

The Lab Gear books

As I've come across errors, I've created corrected PDFs of those pages. (Alas, there's nothing I can do about the error on the cover of one of the books!)

Algebra: Themes, Tools, Concepts

On this page, in addition to corrections, I've included teacher notes to complement the ones in the Teacher's Edition of the book.

Geometry Labs

This is the download page for Geometry Labs, but if you scroll down, you'll find links to Connections, Corrections, Extensions, and Revisions, some of which were suggested by users of the book.

If you find mistakes that I have not yet addressed in those pages, or if you have ideas you'd like to share with other users of the books, please let me know! Thanks in advance.